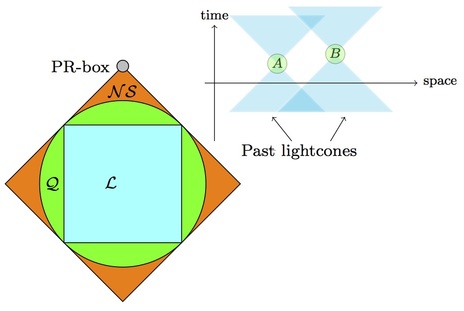
Nonlocality and Quantum Foundations

Bell-nonlocality refers to the intriguing fact that certain correlations between spacelike-separated measurement outcomes cannot be explained by any local common cause. That this phenomenon follows from the prediction of quantum theory was hinted in the well-known paper of Einstein-Podolsky-Rosen in 1935 and made obvious by Bell in 1960's. Since then,  the phenomenon has been verified by increasingly complicated experiments under various stringent conditions. Here, we strive to understand this phenomenon better by working within the generalized theory of nonlocality. 

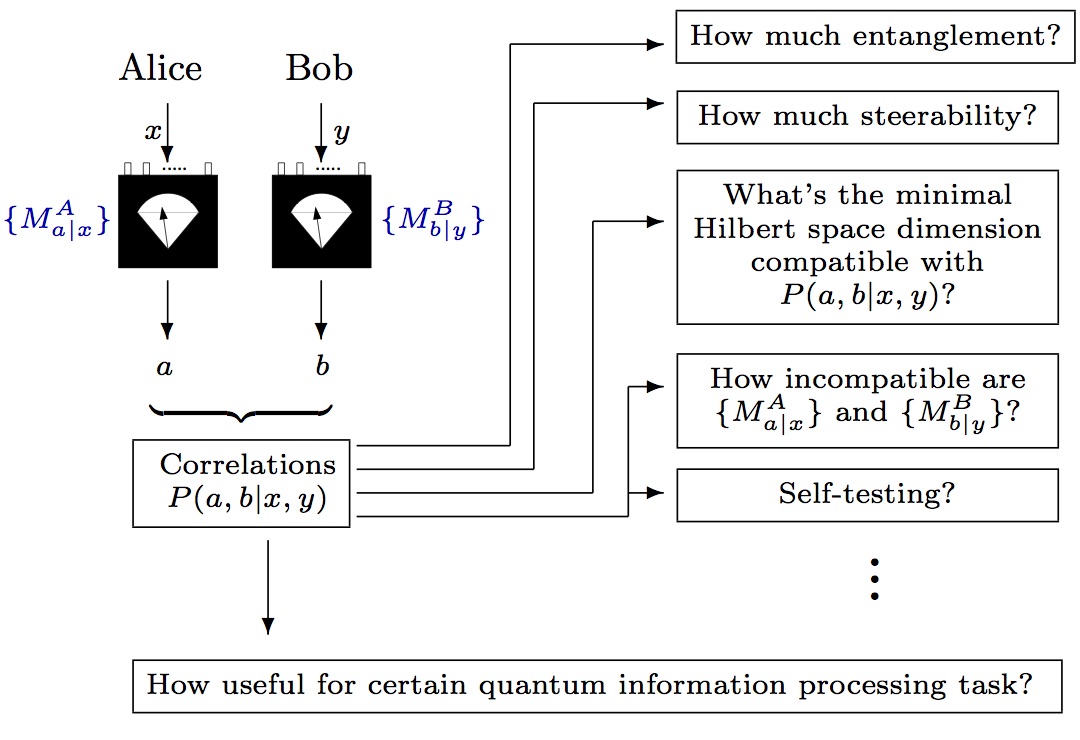
Device-independent Quantum Information

Nonlocal quantum correlations are not only intriguing, but also serve as an indispensable resource for device-independent (DI) quantum information processing. Within the DI paradigm,  conclusions are drawn directly from the raw data measured without relying on any additional assumption about the preparation nor the measurement devices. Prominent examples of this are the possibility to generate shared secret keys, or random numbers that are provably secure assuming only general physical principles. Here, we are particularly interested in using the observed nonlocal correlations to characterize the underlying state and/or the measurement devices.

Quantum vs Classical & Useful Quantum Effects

Quantum theory offers not only nonlocal correlations, but also other bizarre features that do not seem to fit well with our intuition. For instance, our everyday experience is that reading off the time from a clock does not stop it from ticking, but quantum theory dictates that any observation (interaction) with a quantum system necessarily perturbs its evolution. The quantum zeno effect in particular forbids the state from evolving if the system is being measured all the times. How do we reconcile this "disturbing" feature of quantum theory with our everyday experience? Meanwhile, quantum information has taught us that quantum effects can also serve as powerful resources. Our goal here is thus to identify features of the theory that are fundamentally different from our classical intuitions, and in particular quantum effects that are useful, for instance, for future technologies.